In Exponent Basics we worked with whole number exponents. The whole numbers are the set of numbers {0, 1, 2, 3, 4, ...}. The whole numbers are the positive integers, plus zero.
On this page, we will be examing exponents that are
negative integers. {..., -5, -4, -3, -2, -1}.
Negative Integer Exponents |
An value raised to a negative exponent is equal to the number one divided by the value with the sign of the exponent changed to positive.

|
 |
|
For any non-zero number x, and for any positive integer n,
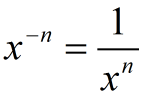 |
There are three important concepts at work in this Rule:
For any non-zero number x, and for any positive integer, n:
Remember, any number (or expression) with a negative exponent ends up
on the opposite side of the fraction bar, with a positive exponent.
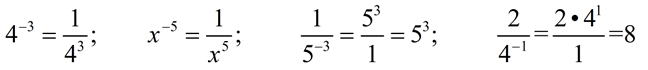
The use of a positive exponent is an application of repeated multiplication by the base:
43 = 4 • 4 • 4 = 64.
The use of a negative exponent produces the opposite of repeated multiplication.
It can be thought of as a form of repeated division by the base:
4-3 = 1 ÷ 4 ÷ 4 ÷ 4 = 0.015625

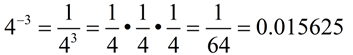
Examples:
1. 
The negative 1 exponent indicates that the value is the same as 1 over 3 raised to a power of positive 1. |
2. 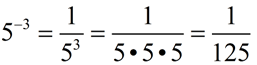
|
3. 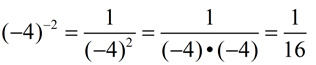
Be sure of keep the negative base in the set of parentheses to avoid calculation errors. |
4. 
This example is working with a decimal base. The same process applies. |
5. 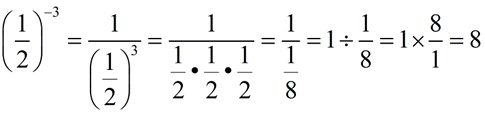
Working with a fraction as the base can be more complicated. When applying the process for negative exponents, a "complex" fraction is formed (a fraction within a fraction). Remember that the fraction bar means divide, when rewriting the complex fraction.
|
6. 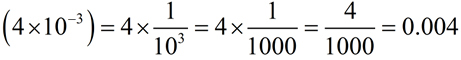
This is similar to scientific notation, which would be 4.0 x 10-3.
|
7. 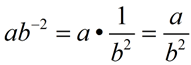
Negative exponents can be also used with variables. Just imagine the variable to be a numeric value and apply the process for negative exponents. |
|
Let's take a closer look at why this Rule is true:
One of the Laws of Exponents is that xm • xn = xm+n.
"When multiplying exponential expressions, if the bases are the same, add the exponents."
If we apply this law to work with a negative exponent, we get 43 • 4-3 = 43+(-3) = 40 = 1.
This application shows us that
43 • 4-3 = 1, which means that 4-3 must the multiplicative identity of 43. Therefore, 4-3 must be a fraction and it must be the reciprocal of 43.
Consequently,  . .
|