There are a wide variety of applications using percents. Many of them are straight forward applications of the methods we have been using to work with percents.
 |
A nursery has 480 evergreen trees, and 75% of the trees are Norway Spruce. How many of the trees are Norway Spruce? |
Solution:
Let x = the number of Norway Spruce
x = 75% of 480
x = 0.75 • 480
x =
360 trees
Method: Finding percent of a number. |
|
|
Percent Increase or Decrease |
There are also situations that involve the concept of "increasing" or "decreasing" an amount by a certain percentage. These problems often involve "money", but may also apply to other situations. In this section, we will deal with situations that do not involve money.
Situations with money will be seen in the next section.
A percent of increase (or decrease) is a ratio of the amount of increase (or decrease) to the original amount.
To find the percent of increase (decrease):
Determine the amount of increase (or decrease).
Divide this result by the given original amount.
Change this decimal value to a percent.
This is the percent of increase (or decrease).
 Find % increase. Find % increase. |
 |
| The number of people attending the first basketball game of the season was 840. The number of people attending the last basketball game of the season was 1200. What was the percent increase in attendance, to nearest percent? |
Solution:
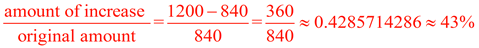
The percent increase in attendance was 43%. |
|
Not all questions ask you to find the percent of increase (decrease):
Some questions GIVE you the percent of increase (decrease).
Read carefully!
The solutions shown below, use an equation method to set up the problem.
 INCREASE
INCREASE |
The population of penguins on Gourdin Island is estimated to be 14,200 pairs. If this number of pairs grows (increases) by 5%, how many pairs will be on Gourdin Island? |
Solution:
First find the number of penguin pairs by which the population will increase.
Let p = number of new pairs
p = 5% • 14200
p = 0.05 • 14200 (changing 5% to a decimal)
p = 710 new pairs of penguins
To find the number of pairs now on the island, ADD the new penguin pairs to the pairs already on the island.
710 + 14200 = 14910 pairs of penguins |
|
|
Be sure to add the percent of increase to the original amount
to find the new total which includes the increase. |
 DECREASE
DECREASE |
A 100 x 200 foot rectangular riding area is fenced off inside of a horse pasture. The creation of this riding area will reduce the pasture area by 10%. What was the area, in square feet, of the pasture before the creation of the riding area? |
Solution:
The area of the riding area is 100 x 200 = 20,000 square feet.
Let x = the original pasture's area
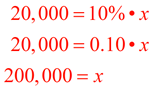
Answer: The original pasture had an area of 200,000 square feet. |
|
|

|
If I increase a value by 20%
and then decrease that amount by 20%,
do I end up back at my starting value?
Despite what you may think, the answer is NO!
Start with 160 and
increase it by 20%.
160 + (20% of 160) = 160 + 32 = 192.
Now, decrease this amount by 20%.
192 - (20% of 192) = 192 - 38.4 = 153.6
160 ≠ 153.6
|
|